by M4t_6_onL | Jul 17, 2021 | Number theory, Set theory
The prime natural numbers are those which have no divisors other than 1 and themselves. They exist in infinite number by Euclid’s theorem, which is not difficult to prove. 1.Prime numbers 1.1.Divisors and primes A prime number is a non-zero natural number (see...
by M4t_6_onL | Jul 17, 2021 | Functions, Set theory
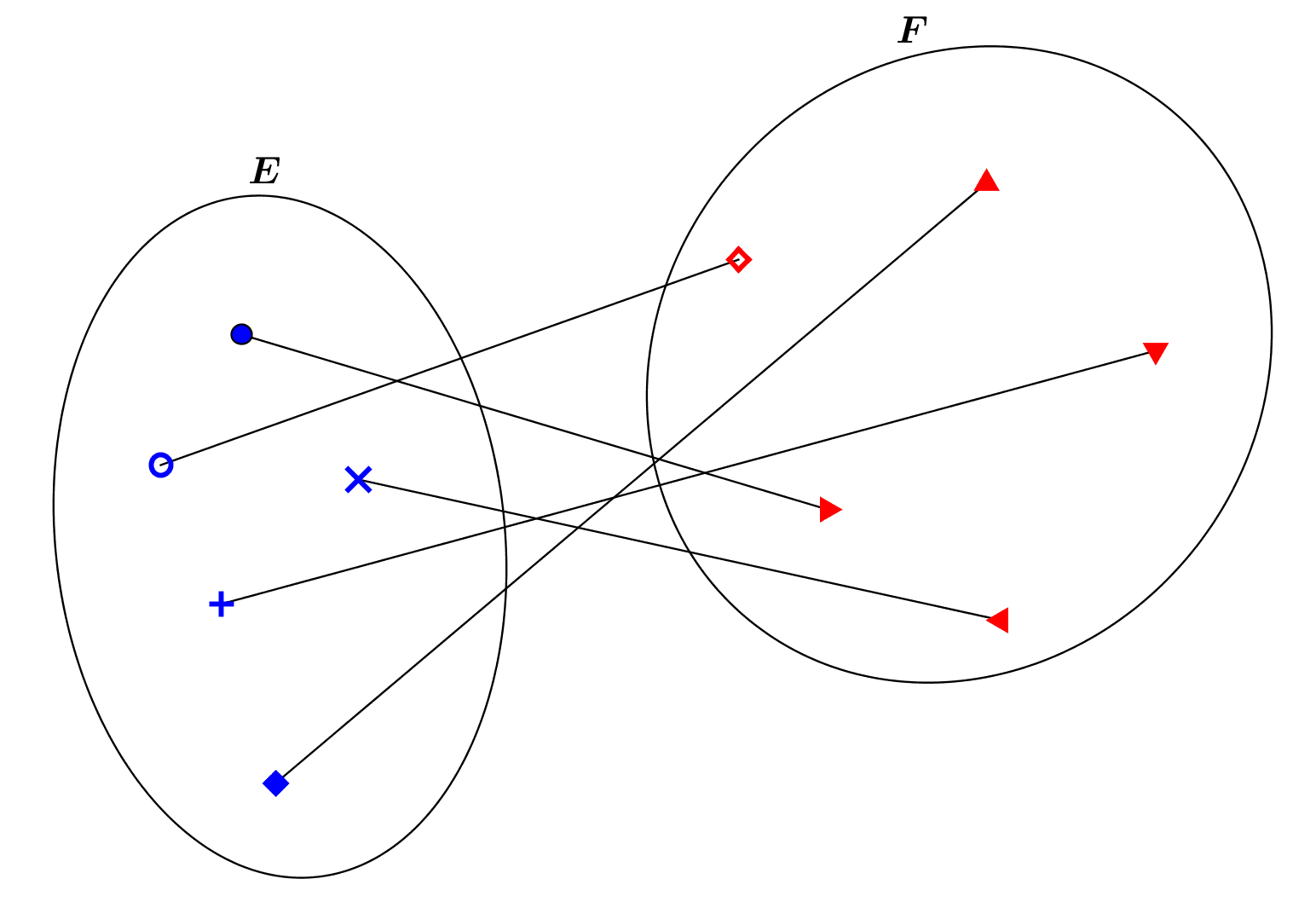
A finite set is a set that can be counted using the natural numbers \(1,\ldots,n\) for a certain natural number \(n\). But what is counting ? And then, what is an infinite set? 1.Comparing sets : the notion of bijection The notions of finite set and infinite set, and...

by M4t_6_onL | Jul 2, 2021 | Set theory
Naive set theory or “potato science” is the natural (and understandable!) foundation of mathematical science. “I know what time is. If you ask me, I don’t know anymore.” Augustine. This insightful quote from Augustine emphasises that...



