
by M4t_6_onL | Jul 10, 2023 | Logic, Number theory, Set theory
Natural arithmetic is the science of natural numbers: it is based on addition, multiplication, natural order and divisibility. Now, all these operations and relations are defined on the basis of the single successor function, whose properties are brought together in...

by M4t_6_onL | Oct 24, 2022 | Algebra, Number theory
Gaussian integers are complex numbers with integer coordinates. Thanks to their norm, a kind of integer measure of their size, we can describe some of their arithmetic properties. In particular, we can determine which are the usual prime numbers that...
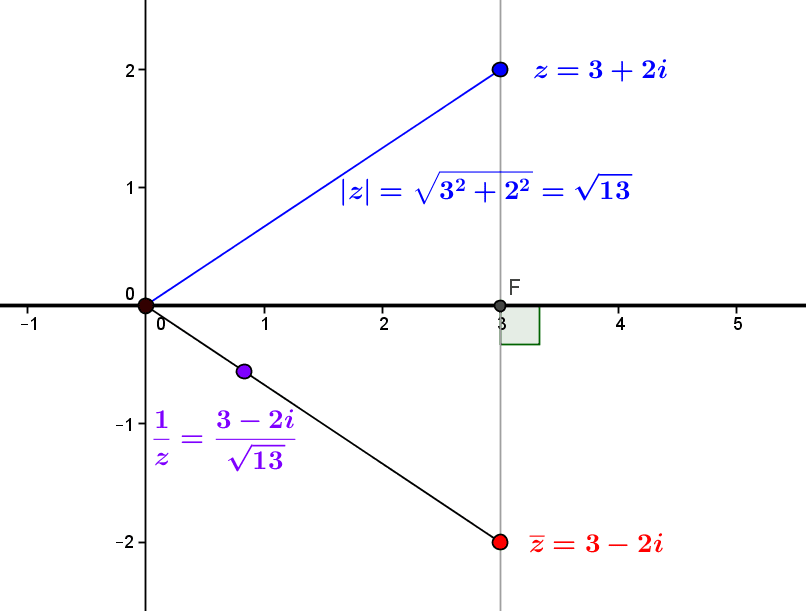
by M4t_6_onL | Aug 1, 2022 | Algebra, Number theory
There are various ways of defining complex numbers. The most direct way is to look at them as points or vectors of the Euclidean plane. Addition and multiplication are then defined using the coordinates. 1. The set \(\mathbb C\) of complex numbers 1.1. A complex...
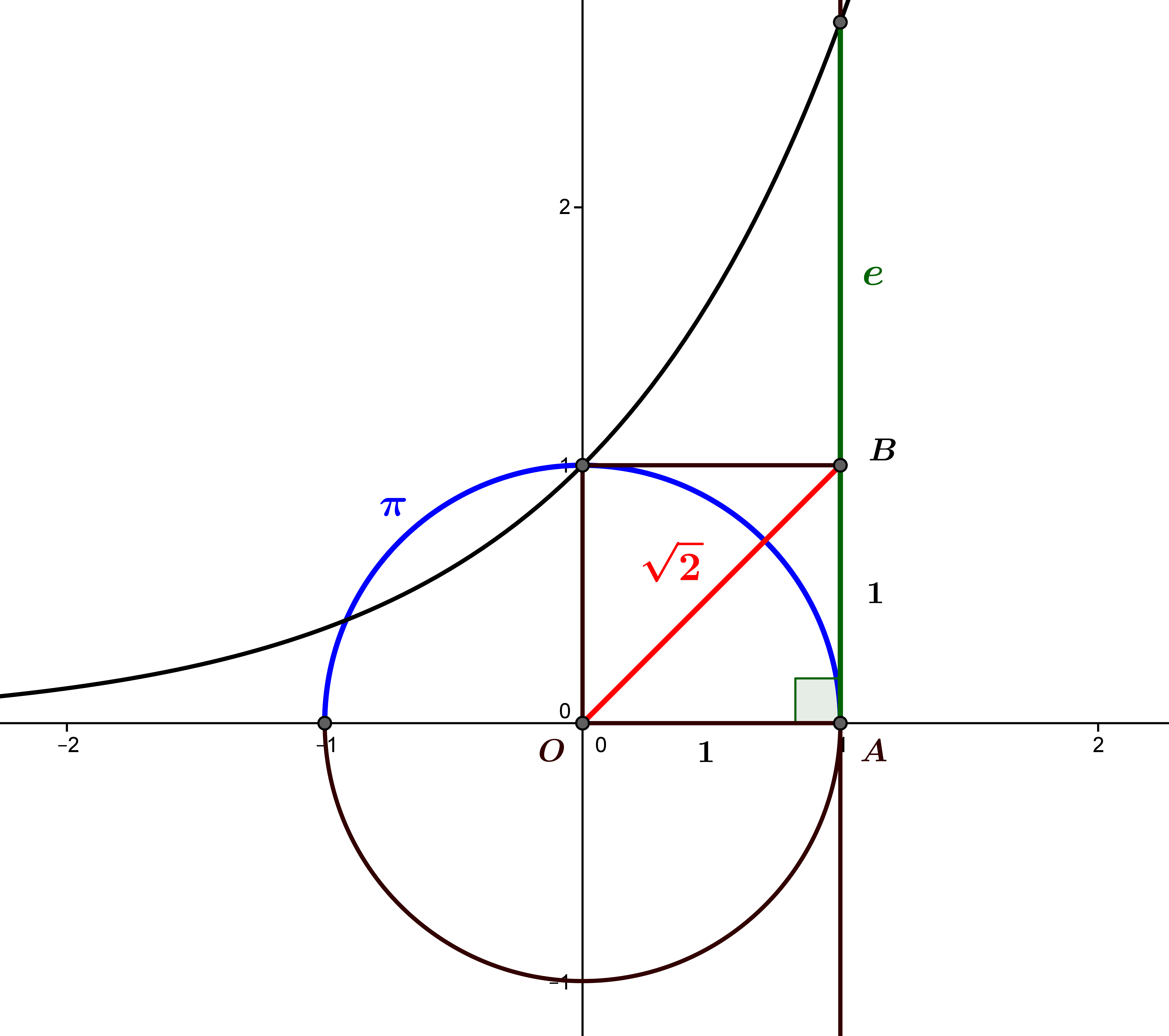
by M4t_6_onL | Aug 15, 2021 | Number theory, Set theory
The real numbers are all the “quantities” that we can order, and we can “construct” them in various ways thanks to set theory. “Numbers govern the world.” Pythagoras The real numbers idealise all the “points” of the...

by M4t_6_onL | Aug 6, 2021 | Number theory, Set theory
1.The intuition of rational numbers Rational numbers, i.e. “fractional” numbers, such as \(-\frac 1 2, \frac{27}{4}, \frac{312}{-6783},\ldots\), form an intuitive set which we note \(\mathbb Q\). It is an extension of the set \(\mathbb Z\) of integers (see...
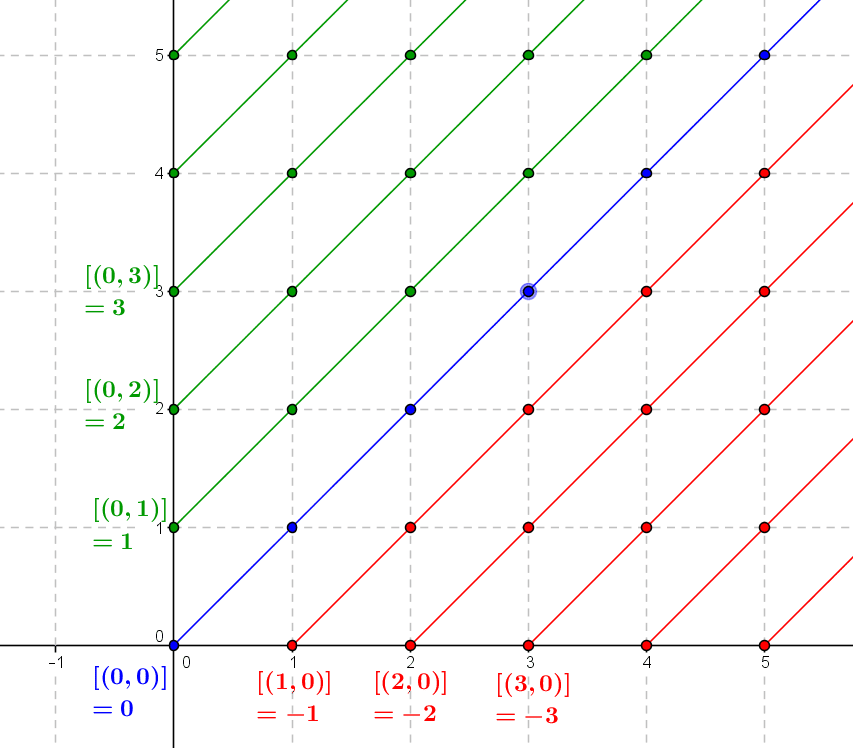
by M4t_6_onL | Jul 30, 2021 | Number theory, Set theory
Integers are an extension of the natural numbers where the existence of subtraction provides a more appropriate framework for certain questions of arithmetic. They can be described axiomatically, but can also be constructed from the set of natural numbers and some...






