by M4t_6_onL | Apr 23, 2022 | Algebra, Geometry
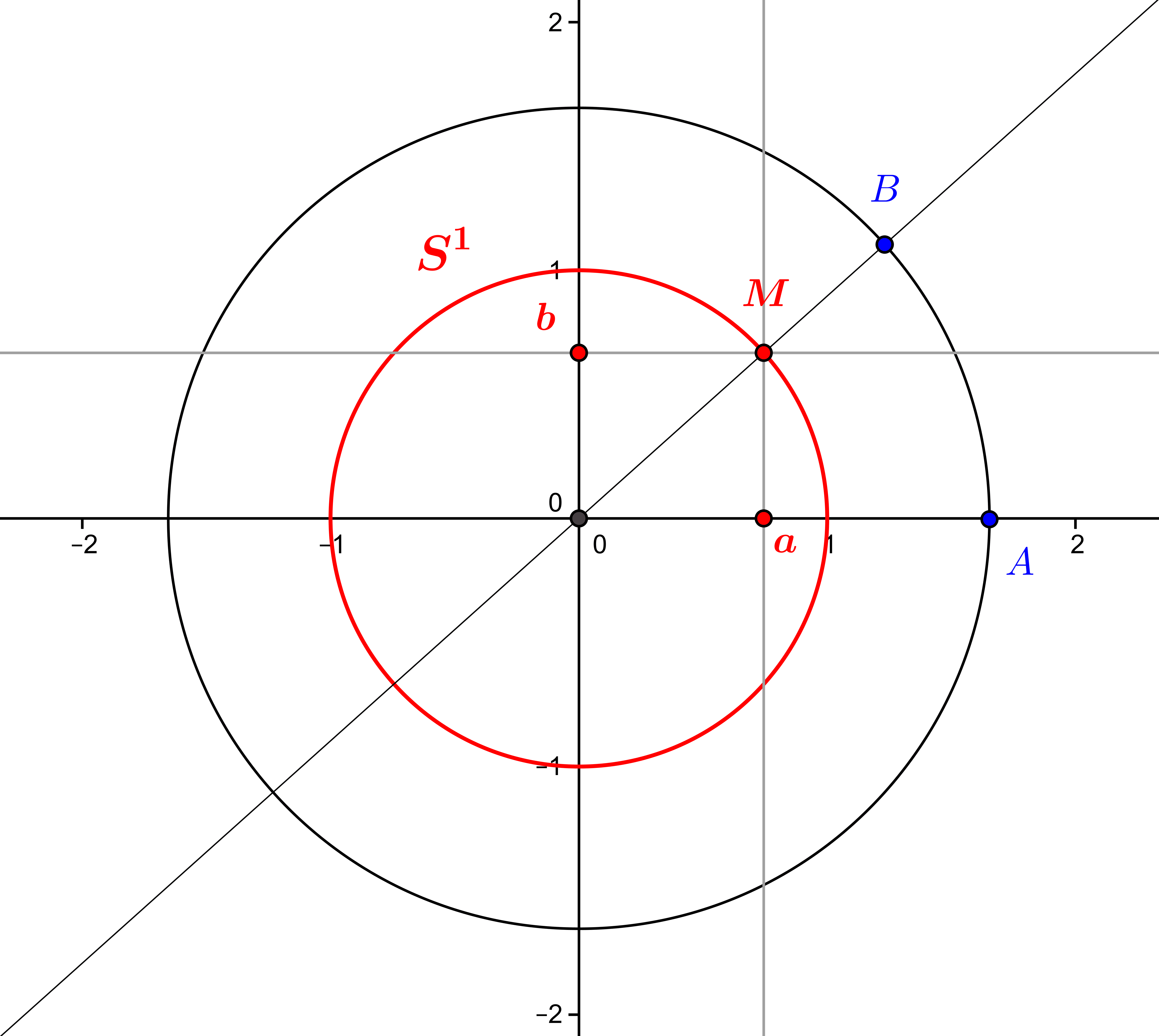
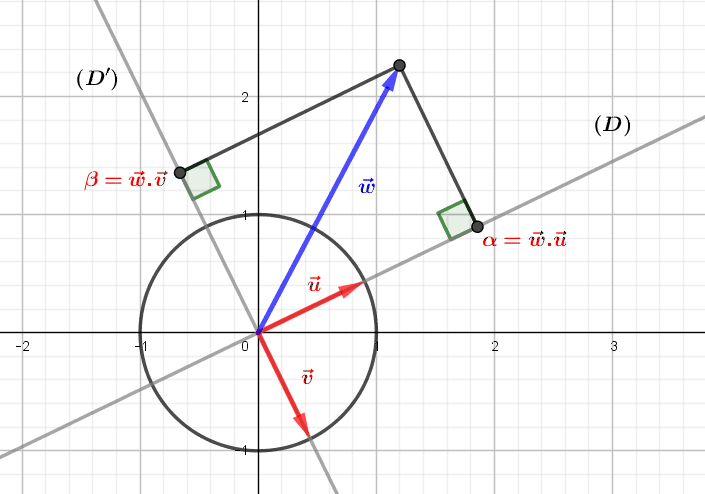
The representation of the Euclidean plane as the Cartesian product \(\mathbb R^2\) allows us to decompose any vector of the plane into two coordinates, its abscissa and its ordinate. This decomposition is linked to a particular and natural “representation...
by M4t_6_onL | Mar 14, 2022 | Trigonometry
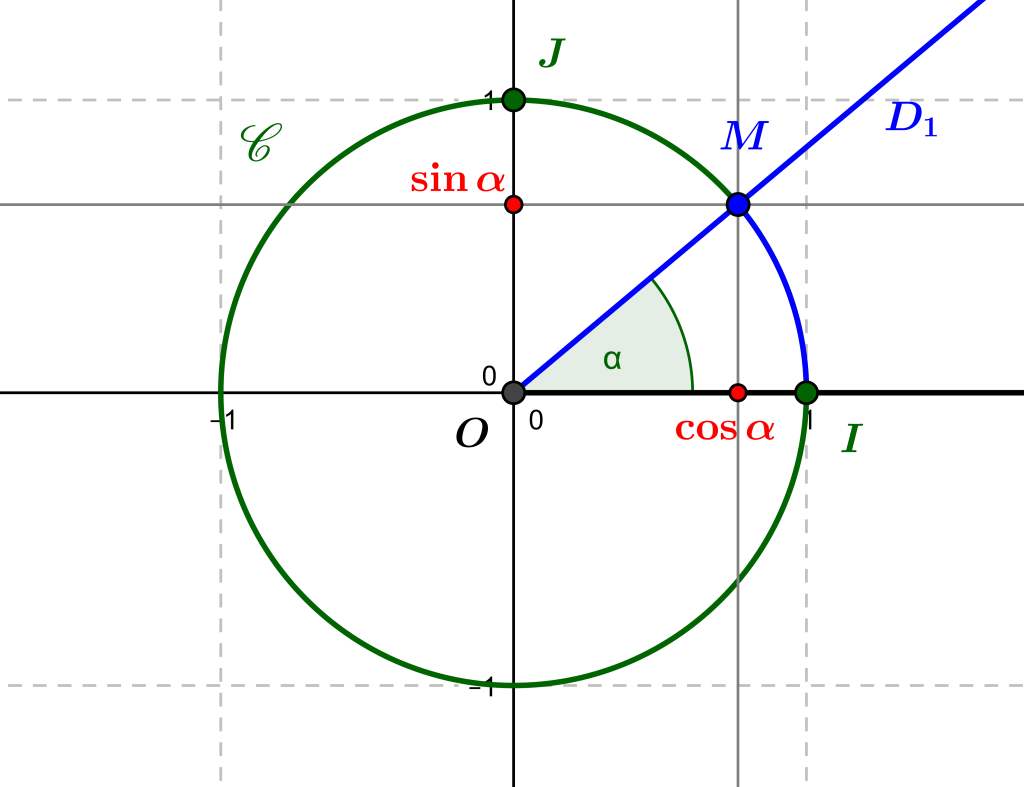
The trigonometric circle allows us to define the cosine, sine and tangent of an oriented angle, and to give an interpretation through Thales’ and Pythagoras’ theorems. Introduction: trigonometry and functions Trigonometry is the study of the relationships...